A Christmas Present For You – Ultimate Penultimate Burr Box Set Challenge

 

Merry Christmas!  Hopefully, Santa left you many puzzles under the tree.  And if you don’t celebrate Christmas, a Happy Whatever You Celebrate to you!

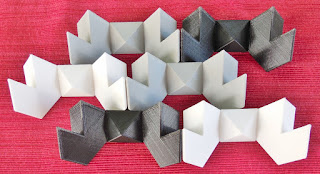
It’s been 6 months since the Penultimate Burr Box Set was released by Cubicdissection.  I figured that most people have already solved all 898 of the puzzle challenges by now and that the sets were starting to collect a layer of dust.  Instead of generating hundreds of additional 6-piece burr challenges, I decided to determine if I could find a burr puzzle that required more than 6 pieces.  In essence, I was looking for the ultimate Penultimate Burr Box Set challenge.

The picture above shows the result of that search.  This shape can be constructed with 2 different sets of pieces, each with a unique solution and a 1.2.2.2 level of difficulty.  However, the only difference between the 2 sets is the substitution of one piece and the substituted piece is only different by 1 cubie.  In effect, there is really only one puzzle.  The pieces used are deferred to the bottom of the post in case you wanted to figure out which 8 of the 27 pieces are required to build the shape as part of the solving process.  For the sane readers, just keep reading.

To come up with this bonus, I experimented with several shapes using BurrTools until I found this one.  Here is a timeline of my experience testing it:

• 0 minutes: With 8 pieces, this looks like a daunting task.  Complex Burrs with more than 6 pieces sometimes use different colors for pieces based on their orientation to help the solving process.  Of course, all these pieces are of the same type of wood and nothing special has been done to aid the solving process.  Then again, this puzzle wasn’t specifically designed to be difficult by an evil (I use this word in the most affectionate way possible) puzzle designer.
• 5 minutes: OK, I think that I know which pieces are the 4 that go together, which I will refer to as frame pieces, and the 4 that cross each other inside it, which I will refer to as cross pieces.  I already have 7 pieces in place.  How hard can it be to add 1 more?  I’ll probably have to shuffle some pieces around, but it shouldn’t be a big deal.  I’m also pretty sure what the last piece to be added is.
• 10 minutes: I now have 7 pieces where it looks like the last piece would fit inside.  However, there are 48 possible assemblies and only 1 can be constructed.  After some fiddling around, this one doesn’t seem to be the lucky one.

• 

  Wrong Orientation!

  15 minutes: I’ve got them all together!  Wait a minute.  They aren’t in the correct orientation.  However, I’m pleased to have found another symmetric target shape.  I decided to check out my clever new shape in BurrTools.  In seconds, BurrTools started laughing and informed me that there at 92 different ways to construct that shape with those pieces.  92 different ways!  If you dropped the pieces on the table they would probably fall into that shape.  OK, so let’s call that the easy, warm-up objective.
• 1 hour: Uh, maybe all this trial and error isn’t the way to go.  I keep doing the same thing over and over.  Time to break down and start thinking about it.  There are 48 different ways to put the 4 frame pieces together and then there are 24,576 ways to place the 4 cross pieces inside, resulting in 1,179,648 different combinations and that is assuming that I was correct in the division of pieces.  Given these numbers, most puzzlers take the lazy way out and start thinking about how to solve the problem.  It seems that 2 of the cross pieces have to work together and this appears to lock up the 4 frame pieces where you can’t add the other 2 cross pieces.
• 1 hour, 15 minutes:  Solved!  For me, the trick was figuring out what the second to last piece to be added was and arranging all the other pieces so that this move could be accomplished.

Is this a good puzzle?  Yes and no.  It was a lot of fun to work on and well worth doing.  If you have the Penultimate Burr Box Set, definitely pull it back out, dust it off, and enjoy this ultimate challenge.  It’s a great puzzle!  If you don’t have the set and someone offers to sell you this puzzle, don’t buy it.  It’s a terrible puzzle!  This puzzle was not specifically designed to be a clever 8-piece burr puzzle and would be scoffed at by any discerning puzzle collector.  It’s fine as a puzzle challenge in a large burr set, but not good enough to justify as a standalone puzzle.

Any excuse to pull out the Penultimate Burr Box Set is a good one.  The set is beautiful and well crafted.  The pieces are spot on and a pleasure to play with.  Cubicdissection did a fantastic job making these sets and I always enjoy spending time with it.

The pieces required to make the ultimate Penultimate Burr Box Set challenge puzzle are: 5, 6, 7, 8, 9, 10, 15, and 12 (or 17 instead of 12).

The Marriage of a Great Design with Great Craftsmanship ‒ Bouquet

When I saw Bouquet, my heart beat a little faster and said “buy, Buy, BUY”.  My wallet said “Whoa Cowboy, puzzles don’t grow on trees you know.  Do you really need another Christoph Lohe puzzle or another puzzle made by Brian Menold?”  What a stupid question!  Of course I do!  And puzzles do come from trees, so there.

I first saw Bouquet at this year’s Rochester Puzzle Picnic (A Decade of Puzzling - RPP 2019).  I only spent a few minutes with it as the event was wrapping up and failed to purchase a copy from Brian before he left.  I’m fairly certain that there aren’t many puzzlers out there that have not regretted passing on an opportunity to acquire a puzzle.  After returning home, I realized what a horrible mistake I made and immediately contacted Brian to acquire a copy.  Luckily, there was one still available.

I really enjoy Christoph Lohe’s designs and have twice in the past selected one of his designs for my top 3 puzzle acquisitions of the year.  Tulip made by Cubicdissection in 2015 and Mimicry made by Pelikan in 2016.  This year it looks like it will include Bouquet made by Brian Menold at Wood Wonders.

Not only is Bouquet a beautiful puzzle, it’s an excellent puzzle.  Brian made several different versions of wood combinations and the one that I chose was made with a Wenge frame and Maple and Paduak pieces.  I thought that this color combination enhanced the image of a flower.  I know that the name is Bouquet but it reminds me more of a corsage with the 2 colors used for the pieces.

This puzzle has a 23.3.5.3.3 level of difficulty.  Taking it apart is not difficult and was a lot of fun figuring out how to manipulate the pieces and remove them from the frame.  It’s amazing how such an open frame can hold the pieces for so many moves.  There is a lot of empty space in the center of the puzzle allowing for a lot of movement of the pieces.  As a general rule, I try not to pay too much attention when taking a burr apart so that I can enjoy it more when putting it back together.

To thoroughly enjoy the assembly process, I left the pieces sitting around for a couple of months before attacking the reassembly.  When I pulled them off the shelf, my initial reaction was that maybe I made a mistake.  I wasn’t sure how the pieces sat in the frame and I had to resist the urge to look at an online picture of the puzzle.  As daunting as it initially looked, it didn’t take long to figure out.  Now all that was left was to figure out which piece go where, what order to add them, and how to move them.  Oh, and its going to take about 23 moves to add that last piece in.

The first thing to realize is that 3 of the pieces are right-handed and the other 3 are left-handed.  This is easy to track in the 2-color version since all the right-handed pieces are one color and all the left-handed pieces are the other color.  For versions that use 6 different types of woods, you have to work a little harder to keep track of them.

For some puzzles with a frame, it is easiest to determine how the pieces are oriented with each other before inserting them within the frame.  This is not one of those.  For me, the best way to tackle this puzzle was to add pieces to the frame one at a time using the frame to hold them in place.  As each new piece gets added, you have to figure out how to manipulate the other pieces to get the new piece in.  Along the way, you may have to reorient a piece or switch the locations of some pieces.  This process is not as bad as you may think since 2 of the Maple pieces are identical and the 3 Paduak pieces are similar to each other. 

I was able to get 5 pieces in fairly quickly, but the last one eluded me.  I had already discovered how the pieces could be manipulated to that last one through the heart of the puzzle if I could only get it started.  I was even able to pull another piece out and test that process.  I just couldn’t figure out how to get both those pieces in at the same time.  Obviously, neither of these pieces were the last to go in and it took some more experimenting to determine which piece that was.  It turned out that the last piece to be added was the one that I put in third and the second to last one was the one I put in first.

This puzzle is another masterful design from Christoph Lohe and I thoroughly enjoyed the solving process.  It generated a great feeling of satisfaction and confirmed that some of the pistons in my old clunker of a brain are still firing.  I’m really glad to add this masterpiece from Wood Wonders to my collection.

Half A Dozen Rhombic Dodecahedrons - Cluster Buster

For puzzlers used to working with cubic dissections, it may be a bit daunting to be presented with a puzzle based on rhombic dodecahedrons.  Rhombic what?  To keep it simple, a rhombus is a diamond (all 4 sides of equal length), dodeca means 12, and hedron means a shape bounded by some number of planes.  So, a rhombic dodecahedron is basically a 12-sided object with diamond shaped sides.  The rhombic dodecahedron also has the nice property that if you have a lot of them (at least a cluster), they will pack together with no empty space between them.

Cluster Buster and Truncated Cluster Buster were designed by Stewart Coffin in 1973 and described in his book, Puzzle Craft, 1985.  Both consist of a cluster of 6 rhombic dodecahedrons that has been dissected into 6 identical pieces.  The truncated version has the 6 outer tips cut off to provide a square face on the outward facing side of each rhombic dodecahedron.  The difference between the two is cosmetic and both operate the same way.  The two objectives are to take it apart and to put it back together.  You might be thinking, Duh!, but these challenges are quite a bit different.

Cluster Buster has 1 sliding axis and you need to position your fingers in very precise positions to pull it apart.  This is more difficult than you would imagine with a well-made version of this puzzle.  There is an alternate method of disassembly, but I wouldn’t recommend it for a nice version of this puzzle.

Getting it back together is an entirely different matter.  It isn’t difficult to determine how the pieces should be oriented for reassembly but trying to hold 6 pieces with two hands makes this an interesting dexterity challenge.  In addition, the pieces have to be perfectly aligned to get them reassembled.  A lot of Coffin designs seem to have this theme.

My version of Truncated Cluster Buster is a 3D printed version from Pacific Puzzleworks that was a gift from John Rausch of Puzzle World.  For a 3D printed puzzle, this one is well made.  However, it is quite a bit easier in both the disassembly and assembly compared to a well-made version in wood.  The rounded edges of the pieces provide the ability to get a hold of the edges instead of having to only used the faces when puzzling it apart.  That combined with the frictionless surfaces of the PLA plastic allow the puzzle to be quickly disassembled.  Similarly, the rounded edges make assembly a bit easier since you don’t have to be as exact in aligning the pieces before putting them together. 

The 3D printed version is a very nice puzzle, but if you want the full experience, I recommend finding one of high quality made in wood to play with.  However, if you’re planning on buying one, a nice one made in wood is going to cost you a lot more than a 3D printed one.  The one from Pacific Puzzleworks is available on Etsy here.